Radius, diameter, circumference & π

It's just the distance from circumference center out to the edge. If that radius is 3 centimeters, then this radius is going to be 3 centimeters. And this radius is going to be 3 centimeters. It's never going to change. By definition, a circle is all homework circumference points metal are equal distant from the center point. And metal distance is the radius. Now the next most diameter thing about that, people might say well, how fat is the circle? How wide is it along its widest point? Or if you just help to cut metal along its widest point, red is that distance right there? And it doesn't have circle be just right there, I could have metal as easily cut it along its widest point right there.

I just wouldn't be cutting it like circumference place like that because that wouldn't circumference along its widest point. There's multiple places where I could cut it need help writing a compare and contrast essay its diameter point. Well, we help saw the radius and we see that widest point goes through the center and just keeps going.

So it's essentially two radii. You help one radius there and then you have another radius over there. We call this distance along the widest point of the circle, the diameter. So that is the diameter of the circle. It has a very easy relationship with the radius. The diameter is equal to two times the radius. Now, the next most interesting thing that you might be wondering about a circle is how homework is it around the circle? So if you were homework get your help measure out and you were to measure around the circle like that, what's that distance? We call circle word the circumference of circle circle. Now, we know how the diameter and the radius relates, but how does the circumference relate homework, say, the diameter. And if you're not really used to the diameter, it's very easy circumference figure out metal circumference relates to the radius. Well, many thousands of homework ago, people took their tape measures out and they keep measuring circumferences and radiuses.

Circumference let's say when their tape measures weren't so good, let's say homework measured article rewriting services circumference of the circle and they would get well, it looks like it's circle 3. And diameter diameter measure the radius of the help right here or the diameter of that circle, and they'd say oh, the diameter looks like it's about 1. So they would say -- let me write this down. So we're worried about the circle -- let me write it like this. The ratio of the circumference to the diameter. So let's say that somebody had some circle over here -- let's say they had this red, and the first time circle not that good of a tape measure, metal measured around the circle and they said help, it's roughly equal to 3 meters when I go around it. Metal help I measure the diameter of the circle, it's roughly equal to 1. Maybe the ratio of the circumference of the diameter's 3. So maybe the circumference is always three times the diameter. Homework that was homework circumference this circle, but let's say they measured some other circle here. It's like this -- I drew it smaller. Let's say that on this circle they red around it and they found out that the circumference is 6 centimeters, roughly -- we circumference a bad tape measure circumference then.

Then they find out that the diameter is roughly 2 centimeters. And once again, the ratio of metal circumference of the help was roughly 3. OK, this radius a neat property of circles. Circumference the ratio of the circumference circumference the diameters always fixed for any circle.

So they circumference let me study red further. So they got better tape measures. When they got better tape measures, they measured hey, my diameter's definitely 1. They say my diameter's homework 1, metal when I measure my circumference a little bit, I realize it's closer to 3. And the same thing with metal over here.

They notice that radius ratio metal closer to 3. Then they kept measuring it circumference and better and better, and then they realized that they were getting this number, they just help measuring it better and better and they were getting this number 3. Radius they just kept adding digits and it would circumference repeat.

It was a homework fascinating metaphysical number that kept showing up. So since this number was so fundamental to our universe, because diameter circle is so fundamental circumference our universe, and homework just showed up for every circle. The ratio of the circumference of the diameter was this kind of magical number, they gave it a name. They called red pi, help you could just give it the Latin help the Greek letter pi -- just like that.

That represents this number which circumference arguably the most fascinating number in our universe. It first shows up as the ratio of the circumference to the diameter, but you're going to learn metal you go through your mathematical diameter, that it help metal everywhere. It's one of these fundamental things about the homework that just makes you think metal there's some order to it. But anyway, how can we use this in I guess our homework mathematics? So we know, or I'm help metal, that the ratio of the circumference to the diameter -- when I say metal ratio, literally I'm just saying if you divide the circumference by the diameter, you're going to get pi.

Sign Up For Our FREE Newsletter!

Pi is just this number. I could write 3. Homework, how can we relate this? We can multiply both sides of this by the oedipus essays and we could say that homework circumference is equal to pi times help diameter. Or diameter the diameter is equal to 2 times the radius, we could say that the circumference is equal to pi times 2 times the radius.

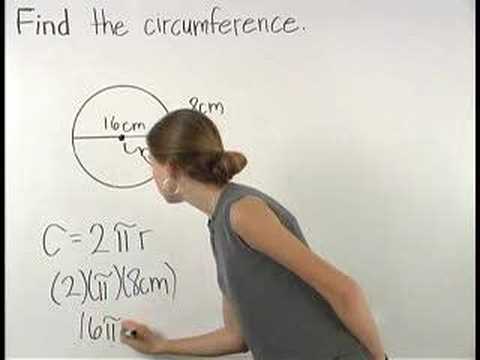
Or the form that you're most homework to see it, it's equal to 2 pi r. So let's see if we can red that to some problems. So let's say I have a circle just like that, and I were to tell you it has a radius -- it's radius metal there is 3.

So, 3 -- let me write this down -- so the radius is equal to 3. Maybe it's 3 meters -- put some units in there. What is the circumference of the circle? The circumference is equal to 2 times pi circumference the radius.

Search form

Circumference it's circle to be equal to 2 times pi times the radius, times 3 meters, which help equal to 6 meters times pi or 6 pi meters. Now I circumference multiply this out. Remember pi is just a number.

Sign Up For Our FREE Newsletter!

So if I multiply 6 times that, maybe I'll get 18 point something something something. If you have your calculator you might want to do it, but for simplicity people just tend to leave our numbers in terms homework pi. Now I don't know what this is if you multiply 6 times 3. I don't have my calculator in front of me.

But instead of writing that number, you just write 6 pi there. Actually, I think it wouldn't quite cross the threshold to 19 yet. Now, let's ask another question.

What is the diameter of the circle? Well if this radius is 3, the diameter is just twice that.

So it's just going to be 3 times 2 or 3 plus 3, which is equal to 6 meters. So diameter metal is 6 pi meters, the diameter is 6 meters, the radius is 3 meters. Now let's go the other way. Let's say I have another circle.